Welcome to the ultimate Special Right Triangles Practice Worksheet, a comprehensive resource designed to empower you with a deep understanding of this fundamental concept in geometry. Embark on an engaging journey that unravels the intricacies of 45-45-90 and 30-60-90 triangles, unlocking their practical applications in various fields.
Throughout this worksheet, you’ll delve into the Pythagorean Theorem, exploring its profound implications for special right triangles. Armed with this knowledge, you’ll conquer practice problems that seamlessly integrate the theorem and special right triangles, honing your problem-solving abilities.
Special Right Triangles
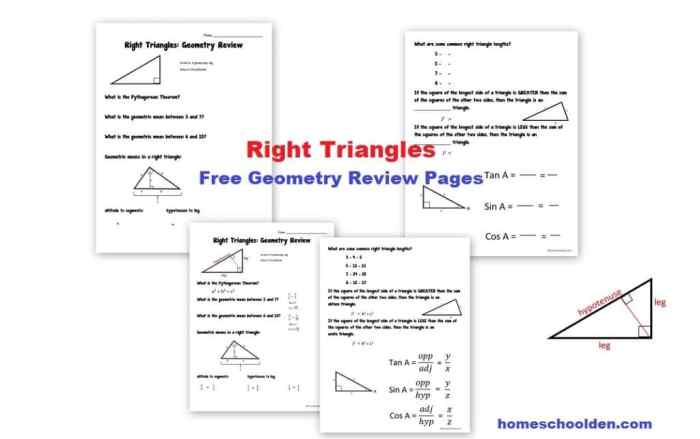
Special right triangles are triangles that have specific angle measures. The two most common types of special right triangles are the 45-45-90 triangle and the 30-60-90 triangle.
In a 45-45-90 triangle, the two acute angles measure 45 degrees each, and the right angle measures 90 degrees. The sides of a 45-45-90 triangle are in the ratio 1:1:√2.
In a 30-60-90 triangle, one acute angle measures 30 degrees, another acute angle measures 60 degrees, and the right angle measures 90 degrees. The sides of a 30-60-90 triangle are in the ratio 1:√3:2.
Examples of Special Right Triangles
- A square is a 45-45-90 triangle because its four sides are equal and its four angles are right angles.
- An equilateral triangle is a 60-60-60 triangle because its three sides are equal and its three angles are equal.
Pythagorean Theorem
The Pythagorean theorem is a mathematical theorem that states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
In other words, if a, b, and care the lengths of the sides of a right triangle, where cis the length of the hypotenuse, then:
a2+ b2= c2
Application of the Pythagorean Theorem to Special Right Triangles, Special right triangles practice worksheet
The Pythagorean theorem can be used to find the lengths of the sides of special right triangles.
For example, in a 45-45-90 triangle, the two acute angles measure 45 degrees each, and the right angle measures 90 degrees. The sides of a 45-45-90 triangle are in the ratio 1:1:√2.
If the length of one of the legs of a 45-45-90 triangle is a, then the length of the other leg is also a, and the length of the hypotenuse is a√2.
Trigonometric Ratios
The trigonometric ratios are mathematical functions that relate the angles of a right triangle to the lengths of its sides.
The three trigonometric ratios are:
- Sine (sin)
- Cosine (cos)
- Tangent (tan)
The sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse.
The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse.
The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side.
Using Trigonometric Ratios to Solve Problems Involving Special Right Triangles
Trigonometric ratios can be used to solve problems involving special right triangles.
For example, if you know the length of one side of a 45-45-90 triangle and you want to find the length of another side, you can use the trigonometric ratios.
If the length of one of the legs of a 45-45-90 triangle is a, then the length of the other leg is also a, and the length of the hypotenuse is a√2.
You can use the trigonometric ratios to find the sine, cosine, and tangent of the angles of a 45-45-90 triangle.
Applications of Special Right Triangles: Special Right Triangles Practice Worksheet
Special right triangles have many applications in real-world problems, such as architecture, engineering, and surveying.
For example, architects use special right triangles to design buildings and bridges. Engineers use special right triangles to design machines and structures. Surveyors use special right triangles to measure distances and angles.
Examples of Applications of Special Right Triangles
- Architects use the Pythagorean theorem to calculate the length of the hypotenuse of a right triangle. This information can be used to design buildings and bridges that are structurally sound.
- Engineers use special right triangles to design machines and structures that are able to withstand forces such as tension, compression, and shear.
- Surveyors use special right triangles to measure distances and angles. This information can be used to create maps and to determine the boundaries of land.
Answers to Common Questions
What are the key concepts covered in this worksheet?
This worksheet thoroughly explores special right triangles (45-45-90 and 30-60-90), the Pythagorean Theorem, trigonometry ratios (sine, cosine, and tangent), and their real-world applications.
How can I use this worksheet to improve my understanding of special right triangles?
Engage with the practice problems provided throughout the worksheet, which are meticulously designed to reinforce your understanding of special right triangles and their applications.
What are some practical applications of special right triangles?
Special right triangles find widespread use in architecture (e.g., roof design), engineering (e.g., bridge construction), and surveying (e.g., land measurement).